The general theory of relativity, developed by Albert Einstein, explains how mass causes the fabric of space and time, or spacetime, to curve. The approach recasts gravity as a curving of spacetime.
Even though this theory is crucial to our understanding of the universe, physicists assert that it may not be the final word. Instead, they contend that theories of quantum gravity, which aim to combine general relativity and quantum physics, hold the key to understanding the most fundamental principles underlying the operation of our universe.
The powerful collisions between black holes, where gravity is most intense, are one site to look for signs of quantum gravity. Colliding black holes roil space-time around them, sending ripples called gravitational waves outward in all directions.
The National Science Foundation-funded LIGO, managed by Caltech and MIT, has now described new methods for putting general relativity to even more stringent tests. Scientists are searching for minor deviances from general relativity that might suggest the existence of quantum gravity by closely examining the structures of black holes and the ripples in space-time they cause.
Yanbei Chen (Ph.D. 03), a professor of physics at Caltech and a co-author of both studies, said, “When two black holes merge to produce a bigger black hole, the final black hole rings like a bell. The quality of the ringing, or its timbre, may be different from the predictions of general relativity if certain theories of quantum gravity are correct. Our methods are designed to look for differences in the quality of this ringdown phase, such as the harmonics and overtones, for example.”
In one of the two investigations, researchers presented a single novel equation to characterize the ringing of black holes in the context of specific quantum gravity theories or the so-called beyond-general-relativity regime.
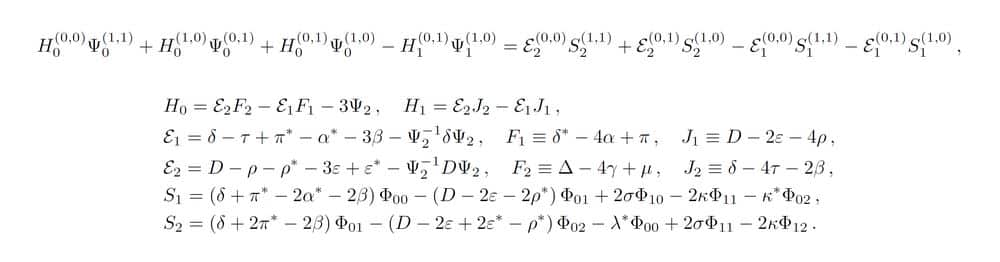
The research expands on an innovative equation created 50 years ago by Caltech’s Robinson Professor of Theoretical Astrophysics Saul Teukolsky (Ph.D. ’73). To comprehend how ripples of space-time geometry propagate around black holes, Teukolsky created a difficult equation.
Dongjun Li, a graduate student at Caltech, said, “If one wants to solve all the Einstein equations of a black hole merger to simulate it accurately, they must turn to supercomputers. Numerical relativity methods are incredibly important for accurately simulating black hole mergers, and they provide a crucial foundation for interpreting LIGO data. But it is extremely hard for physicists to draw intuitions directly from the numerical results. The Teukolsky equation gives us an intuitive look at what is going on in the ringdown phase.”
“Our new equation allows us to model and understand gravitational waves propagating around black holes that are more exotic than Einstein predicted.”
The second study describes a new way to apply Li’s equation to actual data acquired by LIGO and its partners in their next observational run. This data processing method removes characteristics of a black hole’s ringing predicted by general relativity using a series of filters to identify possibly subtle, beyond-general-relativity signs.
Caltech graduate student Sizheng Ma said, “We can look for features described by Dongjun’s equation in the data that LIGO, Virgo, and KAGRA will collect. Dongjun has found a way to translate a large set of complex equations into just one equation, and this is tremendously helpful. This equation is more efficient and easier to use than previous methods.”
Li said, “The two studies complement each other well. I was initially worried that the signatures my equation predicts would be buried under the multiple overtones and harmonics; fortunately, Sizheng’s filters can remove all these known features, which allows us to focus on the differences.”
Journal References:
- Dongjun Li, Pratik Wagle, Yanbei Chen, and Nicolás Yunes. Perturbations of Spinning Black Holes beyond General Relativity: Modified Teukolsky Equation. Physical Review X. DOI: 10.1103/PhysRevX.13.021029
- Sizheng Ma, Ling Sun, and Yanbei Chen. Black Hole Spectroscopy by Mode Cleaning. Physical Review Letters. DOI: 10.1103/PhysRevLett.130.141401