Knots are nightmares, especially for those who have long hair. However, with some practice, most people can detangle their hair with the least amount of pain: start at the bottom and work your way up to the scalp with short, soft brushes, using a detangler.
While brushing his little daughter’s hair, L. Mahadevan, the Lola England de Valpine Professor of Applied Mathematics, Organismic and Evolutionary Biology, and Physics, studied the mechanics of combing.
In a new study, Mahadevan and co-authors Thomas Plumb Reyes and Nicholas Charles explore the mathematics of combing. They explained why the brushing technique used by so many is the most effective method to detangle a bundle of fibers.
They simplified the problem by simulating two helically entwined filaments rather than a whole head of hair. Their model helps them study the detangling of the double helix via a single stiff tine that moves along it, leaving two untangled filaments in its wake.
Plumb-Reyes, a graduate student at SEAS, said, “We measured the forces and deformations associated with combing and then simulated it numerically.”
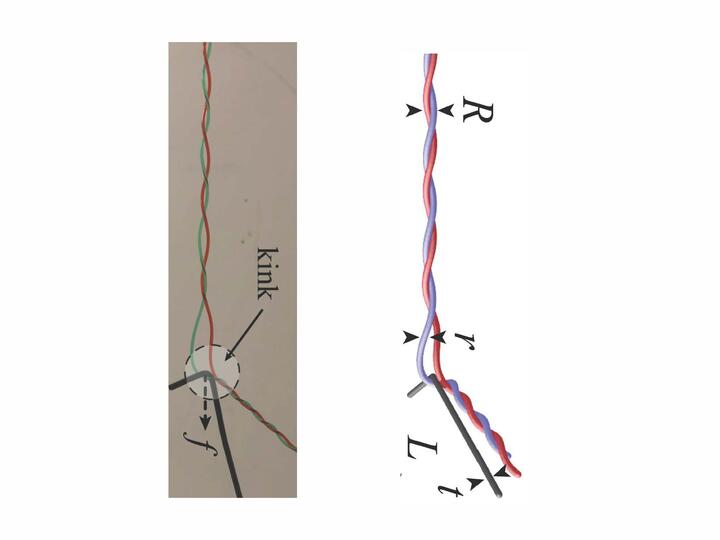
Nicholas Charles, a graduate student at SEAS, said, “Short strokes that start at the free end and move towards the clamped end remove tangles by creating a flow of a mathematical quantity called the ‘link density’ that characterizes the amount that hair strands that are braided with each other, consistent with simulations of the process.”
The team also discovered the ideal minimum length for each stroke – any shorter and combing out all the knots would take an eternity, and it would be too painful any longer.
Professor Daniela Rus and her team at MIT used these mathematical principles of brushing to design algorithms for brushing hair by a robot.
Scientists sought to study the mechanics of brushing curlier hair and how it responds to humidity and temperature with further studies. This may lead to a mathematical understanding of a fact every person with curly hair knows: never brush dry hair.
Journal Reference:
- Thomas B. Plumb-Reyes, Nicholas Charles and L. Mahadevan. Combing a double helix. DOI: 10.1039/D1SM01533H
